Carnot Engine - Cycle, Formula, FAQs

The Carnot engine is a theoretical thermodynamic cycle that represents the most efficient possible engine for converting heat into work. It was proposed by French physicist Sadi Carnot in 1824 and operates between two heat reservoirs at different temperatures—one hot and one cold. The Carnot cycle consists of four reversible processes: two isothermal (constant temperature) and two adiabatic (no heat exchange). Although no real engine can achieve the ideal efficiency of a Carnot engine due to practical limitations like friction and irreversibility, its principles form the foundation for modern thermal power plants and refrigeration systems.

In real life, Carnot's concept is observed in systems like car engines, air conditioners, and refrigerators, where heat is transferred to produce mechanical work or to cool spaces. Understanding the Carnot engine helps engineers design more efficient machines by minimizing energy loss.

What is a Carnot Engine?

Steam engines began to play an increasingly important role in industry and transportation in the early nineteenth century. Reflections on the Motive Power of Fire were published in 1824 by Nicolas Léonard Sadi Carnot (1796-1832), a French military engineer. The book proposed a comprehensive theory of heat engines as well as an idealised model of a thermodynamic system for a heat engine known as the Carnot cycle. Carnot is widely regarded as the "Father of Thermodynamics," having laid the groundwork for the second law of thermodynamics.

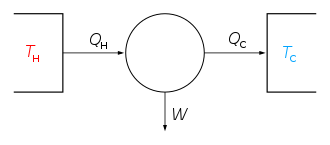
Carnot Heat Engine: Diagram

In the diagram, the "working body" (system), a term used by Clausius in 1850, can be any fluid or vapour body through which heat Q can be injected or transferred to produce work. Carnot proposed that the fluid body may be an expandable substance, such as the vapour of water, the vapour of alcohol, the vapour of mercury, a permanent gas, or air, among others. Although engines came in a variety of layouts during these early years, QH was often supplied by a boiler, wherein water was boiled over a furnace; QC was typically supplied by a stream of cold flowing water in the form of a condenser positioned on a separate section of the engine. W symbolises the movement of the output work. The output work, W, reflects the movement of the piston as it turns a crank-arm, which is then used to power a pulley, which is commonly used to push water out of flooded salt mines. Work, according to Carnot, is a "weight lifted through a height."

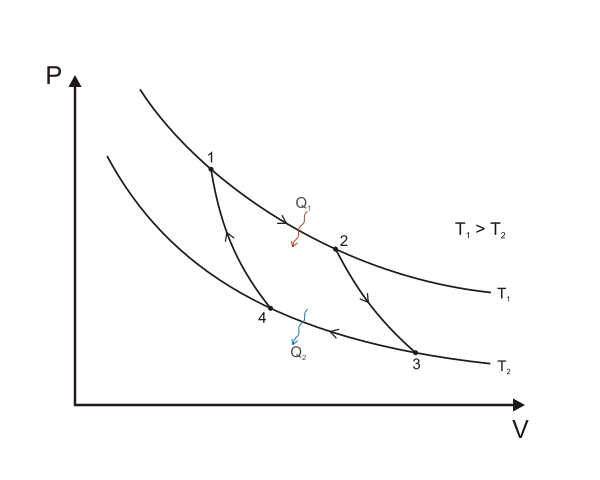
During these Carnot cycle processes, the substance can be expanded and compressed to the required point and then returned to its initial state.

The Carnot cycle is made up of the four Carnot cycle processes listed below:

1. A Carnot cycle process of reversible isothermal gas expansion. The ideal gas in the system receives q_in quantity of heat from a heat source at a high-temperature T_high, expands, and does work on the surroundings in this Carnot cycle process.

2. An adiabatic gas expansion Carnot cycle process that is reversible. The system is thermally insulated throughout this Carnot cycle process. The gas continues to expand and exert influence on its surroundings, causing the system to drop to a lower temperature, T_low.

3. An isothermal gas compression method that is reversible. In this Carnot cycle process, the surroundings do work on the gas at T_low, resulting in a loss of heat, q_out.

4. An adiabatic gas compression method that is reversible. The system is thermally insulated throughout this Carnot cycle process. The surroundings continue to operate on the gas, causing the temperature to increase back to T_high.

Efficiency of Carnot Engine Formula (Carnot Engine Efficiency Formula)

It is the most efficient engine possible based on the premise of no accidental wasteful Carnot cycle processes such as friction and no heat conduction between various sections of the engine at different temperatures. The Carnot efficiency is defined as the ratio of energy output to energy intake.

Efficiency of Carnot engine

$\eta=1-T c / T h$
$\eta$: is the thermal efficiency of the engine
Tc: is the sink temperature
Th: is the source temperature

Recommended Topic Video

Solved Examples Based on Carnot Engine

Example 1: A Carnot engine takes $3 \times 10^6$ cal of heat from a reservoir at $627^{\circ} \mathrm{C}$, and gives it to a sink at $27^{\circ} \mathrm{C}$. The work done by the engine is

1) $4.2 \times 10^6 \mathrm{~J}$
2) $8.4 \times 10^6 \mathrm{~J}$
3) $16.8 \times 10^6 \mathrm{~J}$
4) zero

Solution:

Efficiency of a cyclic process

$\eta=\frac{\text { work done per cyclic }}{\text { gross heat supplied per cyclic }}$

wherein

Gross heat implied only part of heat absorbed.

The efficiency of a Carnot cycle
$
\eta=\frac{W}{Q_1}=1-\frac{T_2}{T_1}
$

$T_1$ and $T_2$ are in kelvin
wherein
$T_1=$ Source temperature
$T_2=$ Sink Temperature

$
\left(T_1>T_2\right)
$
Given,

$
\begin{aligned}
& \eta=1-\frac{T_2}{T_1}=\frac{W}{Q_1} \\
& =1-\frac{300}{900}=\frac{W}{3 \times 10^6 \mathrm{cal}} \\
& \frac{\mathrm{W}}{\text { or } 3 \times 10^6 \mathrm{cal}}=\frac{2}{3} \\
& \text { or } \mathrm{W}=2 \times 10^6 \mathrm{kcal} \\
& =8.4 \times 10^6 \mathrm{Joule}
\end{aligned}
$

Hence, the answer is the option (2).

Example 2: Even the Carnot engine cannot give 100% efficiency because we cannot

1) prevent radiation

2) find ideal sources

3) reach absolute zero temperature

4) eliminate friction.

Solution:

The efficiency of a Carnot cycle
$
\eta=\frac{W}{Q_1}=1-\frac{T_2}{T_1}
$

$T_1$ and $T_2$ are in kelvin
wherein
$T_1=$ Source temperature
$T_2=$ Sink Temperature

$
\left(T_1>T_2\right)
$
For Carnot Cycle

Efficiency

$
\eta=1-\frac{T 2}{T 1}
$
Efficiency will be $100 \%$ only if $\mathrm{T}_2=\mathrm{OK}$

But this is not practically possible.

Hence the answer is the option (3).

Example 3: Two Carnot engines $A$ and $B$ are operated in series. Engine $A$ receives heat from a reservoir at 600 K and rejects heat to a reservoir at temperature $T$. Engine $B$ receives heat rejected by engine $A$ and $\underline{\eta_B}$
in turn rejects it to a reservoir at 100 K . If the efficiencies of the two engines $A$ and $B$ are represented by $\eta A$ and $\eta B$, respectively, then what is the value of $\eta_A$ ? (Work output is same for both)

1) $\frac{12}{7}$
2) $\frac{7}{12}$
3) $\frac{12}{5}$
4) $\frac{5}{12}$

Solution:

Efficiency of a Carnot cycle
$
\eta=\frac{W}{Q_1}=1-\frac{T_2}{T_1}
$

$T_1$ and $T_2$ are in kelvin wherein
$T_1=$ Source temperature
$T_2=$ Sink Temperature

$
\left(T_1>T_2\right)
$

$
\begin{aligned}
& \eta_A=1-\frac{T}{600}=\frac{w}{Q_1} \rightarrow(1) \\
& \eta_B=1-\frac{100}{T}=\frac{w}{Q_2}=\frac{w}{Q_1-w} \\
& \eta_B=1-\frac{100}{T}=\frac{1}{\frac{Q_1}{w}-1}
\end{aligned}
$

from eqn1:

$
\begin{aligned}
& \eta_B=1-\frac{100}{T}=\frac{1}{\frac{1}{1-\frac{T}{600}}-1}=\frac{1-\frac{T}{600}}{\frac{T}{600}} \\
& \eta_B=\frac{600}{T}-1=1-\frac{100}{T} \text { or } \frac{700}{T}=2 \text { or } T=350 \mathrm{~K} \\
& \frac{\eta_B}{\eta_A}=\frac{1-\frac{100}{T}}{1-\frac{T}{600}}=\frac{12}{7}
\end{aligned}
$

Hence the answer is the option (1).

Example 4: Three Carnot engines operate in series between a heat source at a temperature $T_1$ and a heat sink at a temperature $T_4$ (see figure). There are two other reservoirs at temperature $T_2$ and $T_3$, as shown, with $T_1>T_2>T_3>T_4$. The three engines are equally efficient if:

1) $
\mathrm{T}_2=\left(\mathrm{T}_1 \mathrm{~T}_4\right)^{1 / 2} ; \mathrm{T}_3=\left(\mathrm{T}_1^2 \mathrm{~T}_4\right)^{1 / 3}
$

2) $
\mathrm{T}_2=\left(\mathrm{T}_1^2 \mathrm{~T}_4\right)^{1 / 3} ; \mathrm{T}_3=\left(\mathrm{T}_1 \mathrm{~T}_4^2\right)^{1 / 3}
$

3) $\mathrm{T}_2=\left(\mathrm{T}_1 \mathrm{~T}_4^2\right)^{1 / 3} ; \mathrm{T}_3=\left(\mathrm{T}_1^2 \mathrm{~T}_4\right)^{1 / 3}$

4) $\mathrm{T}_2=\left(\mathrm{T}_1^3 \mathrm{~T}_4\right)^{1 / 4} ; \mathrm{T}_3=\left(\mathrm{T}_1 \mathrm{~T}_4^3\right)^{1 / 4}$

Solution:

Efficiency of a Carnot cycle
$
\eta=\frac{W}{Q_1}=1-\frac{T_2}{T_1}
$

$T_1$ and $T_2$ are in kelvin wherein
$T_1=$ Source temperature
$T_2=$ Sink Temperature
$\left(T_1>T_2\right)$

$
\begin{gathered}
\varepsilon_1=1-\frac{T_2}{T_1} \\
\varepsilon_2=1-\frac{T_3}{T_2} \\
\varepsilon_3=1-\frac{T_4}{T_3} \\
\varepsilon_1=\varepsilon_2=\varepsilon_3 \\
\Rightarrow 1-\frac{T_2}{T_1}=1-\frac{T_3}{T_2}=1-\frac{T_4}{T_3}
\end{gathered}
$
$
\begin{aligned}
& \frac{T_2}{T_1}=\frac{T_3}{T_2}=\frac{T_4}{T_3} \\
& \Rightarrow \frac{T_2}{T_1}=\frac{T_3}{T_2} \\
& \Rightarrow T_2=\sqrt{T_1 T_3} \cdots \cdots(1) \\
& \text { SIMILARLY } \\
& T_3=\sqrt{T_2 T_4} \cdots \cdots(2) \text { PUT IN } \\
& T_2=\sqrt{T_1 T_3} \\
& T_{2=}=\sqrt{T_1 \sqrt{T_2 T_4}} \\
& T_2=T_1^{\frac{2}{3}} T_4^{\frac{1}{3}}
\end{aligned}
$

Hence the answer is the option (2).

Example 5: A Carnot engine has an efficiency of $1 / 6$. When the temperature of the sink is reduced $62^{\circ} \mathrm{C}$, its efficiency is doubled. The temperatures of the source and the sink are, respectively,

1) $62^{\circ} \mathrm{C}, 124^{\circ} \mathrm{C}$
2) $99^{\circ} \mathrm{C}, 37^{\circ} \mathrm{C}$
3) $124^{\circ} \mathrm{C}, 62^{\circ} \mathrm{C}$
4) $37^{\circ} \mathrm{C}, 99^{\circ} \mathrm{C}$

Solution:

The efficiency of a Carnot cycle

$\begin{aligned} & \eta=\frac{W}{Q_1}=1-\frac{T_2}{T_1} \\ & T_1 \text { and } T_2 \text { are in kelvin } \\ & \text { wherein } \\ & T_1=\text { Source temperature } \\ & T_2=\text { sink Temperature } \\ & \left(T_1>T_2\right) \\ & \text { given } \\ & \frac{1}{6}=1-\frac{T \sin k}{T \operatorname{sourse}} \\ & \frac{T \sin k}{T \operatorname{sourse}}=\frac{5}{6} \cdots \text { (1) }\end{aligned}$

also
$
\begin{aligned}
& \frac{2}{6}=1-\frac{T \sin k-62}{T \text { sourse }} \\
& \frac{1}{3}=1-\frac{5}{6}+\frac{62}{\text { Tsourse }} \\
& \frac{1}{6}=\frac{62}{\text { Tsourse }} \\
& \text { Tsourse }=372 \mathrm{~K}=99^{\circ} \mathrm{C} \\
& \text { Tsin } k=\frac{5}{6} \times 372=310 \mathrm{~K}=37^{\circ} \mathrm{C}
\end{aligned}
$

Hence, the answer is the option (2).