Parallel Perpendicular Axes Theorem - Statement, Formula, FAQs

Edited By Vishal kumar | Updated on Nov 29, 2024 11:02 AM IST

Theorems on parallel and perpendicular axes are basic theorems used in rotational mechanics to simplify the calculation of moment of inertia. The Theorem of Parallel Axes states how the moment of inertia of the body about an axis can be derived from its moment of inertia about a parallel axis on the other hand, the perpendicular theorem states that the moment of inertia of a body about an axis perpendicular to its plane is equal to the sum of its moments about any two mutually perpendicular axes in its plane which intersect each other at the point where the perpendicular axis pass through it.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

What is a Moment of Inertia?
What is the Parallel Axis Theorem?
Parallel Axis Theorem Formula
Parallel Axis Theorem Derivation
Perpendicular Axis Theorem
Perpendicular Axis Theorem Formula

Parallel Perpendicular Axes Theorem - Statement, Formula, FAQs

What is a Moment of Inertia?

We know that inertia is a property that a body already has, to resist the change in its linear state of motion or state of rest. This is a property that measures the mass of the body. However, the moment of inertia which is denoted by (I) is the measure of property or ability of a body to resist its state of rotational motion. It plays the same exact role in rotational motion as mass plays in linear motion and hence we can draw an analogy between them.

So, we can define the moment of inertia as the property of a body to oppose any change in its state of uniform motion or its state of rest $\mathrm{I}=\mathrm{Mr}^2$, where r is the perpendicular distance of a particle from the rotational axis and M is the mass of the body in rotational motion.

Also, read

What is the Parallel Axis Theorem?

The moment of inertia of a body about an axis that passes through its centre is equal to the sum of that about an axis which passes through its centre, and the product of the mass of the body and the square of the distance between the two axes.

Parallel Axis Theorem Formula

The parallel axis theorem statement can be expressed as follows:

$$
\mathrm{I}=\mathrm{I}_{\mathrm{C}}+\mathrm{Mh}^2
$$
Where,
I is the moment of inertia of the body
$\mathrm{I}_{\mathrm{C}}$ is the moment of inertia about the center
$M$ is the mass of the body
$\mathrm{h}^2$ is the square of the distance between the two axes

Parallel Axis Theorem Derivation

Let $\mathrm{I}_{\mathrm{C}}$ be the moment of inertia of an axis that is passing through the centre of mass (AB from the figure) and I will be the moment of inertia about the axis $A^{\prime} B^{\prime}$ at a distance of $h$.

Consider a particle of mass $m$ at a distance $r$ from the centre of gravity of the body. Then,
Distance from $A^{\prime} B^{\prime}=r+h$

$$
\begin{aligned}
& I=\sum m(r+h)^2 \\
& I=\sum m\left(r^2+h^2+2 r h\right) \\
& I=\sum m r^2+\sum m h^2+\sum 2 r h \\
& I=I_C+h 2 \sum m+2 h \sum m r \\
& I=I_C+M h^2+0 \\
& I=I_C+M h^2
\end{aligned}
$$

Related Topics

JEE Main Highest Scoring Chapters & Topics

Just Study 40% Syllabus and Score upto 100%

Download E-book

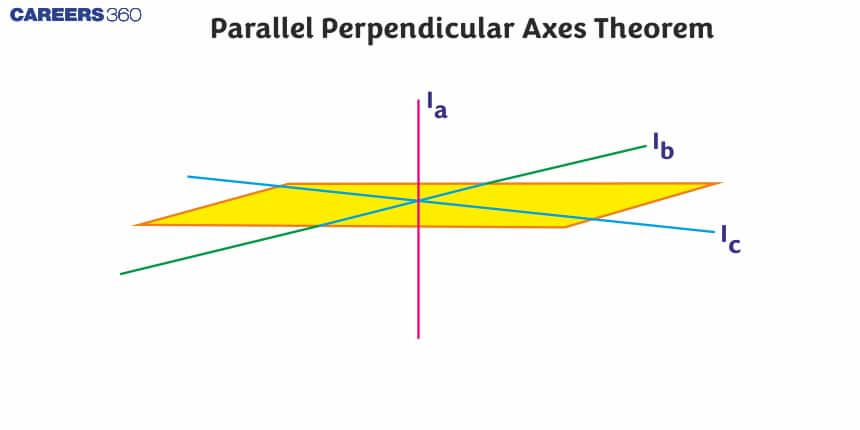
Perpendicular Axis Theorem

The perpendicular axis theorem states that for any plane body, the moment of inertia about any of its axes which are perpendicular to the plane is equal to the sum of the moment of inertia about any two perpendicular axes in the plane of the body which intersect the first axis in the plane.

Perpendicular Axis Theorem Formula

The perpendicular axis theorem is used when the body is symmetric in shape about two out of the three axes. If the moment of inertia about two of the axes is known the moment of inertia about the third axis can be found using the expression:

perpendicular axis theorem

$$
I_a=I_b+I_c
$$

Frequently Asked Questions (FAQs)

1. Find out the relation between I1 and I2, where I1 and I2 are moments of inertia of a rigid body of mass m as in fig-6.

We use the parallel axis theorem- I1 =Icom+ma2 and I2=Icom+mb2

Therefore, I1 - I2=m(a2-b2)

2. Find the moment of inertia of a disc about an axis through its tangent and perpendicular to the plane. (See figure-7)

First we will find moment of inertia about an axis passing through its center and

perpendicular to the plane i.e. Icom

For this, consider an elementary ring of mass dm and width dr at a distance r from the center of

disc. Then, moment of inertia for the elementary ring is- dI=dm.r2

As, dm=MR2.2πrdr

Then, Icom =dIring=0RMR2.2πrdr.r2=MR2/2

Now, to find moment of inertia about axis through its tangent, we use parallel axis theorem-

I=Icom+ MR2, as h=R here.

I=3MR22

3. Find the moment of inertia of a given sphere (uniform) having a mass m and radius R about any tangent if the sphere is a solid.

We use parallel axis theorem, I=ICOM+Mh2

ICOM=25mR2 , d=R here

Therefore, I=75mR2

4. Find moment of inertia of a disc about an axis parallel to its plane and passing through its center of mass (see figure-8).

We already know that, Icom= MR2/2

By perpendicular axis theorem formula, Iz=Ix+Iy

=> MR2/2= 2ID, as the ring is symmetrical, thus Ix=Iy=ID

=>ID=(14)MR2

5. 5. We are given a symmetric lamina of mass M consisting of a square shape with a semicircular section over each edge of the square as in figure-9. The side of the square is given 2a.The moment of inertia of the lamina about an axis through its center of m

Using perpendicular axis theorem-

Ix=Iy

2Ix= 1.6 Ma2

Ix=0.8Ma2

IAB=Ix+M(2a)2=4.8Ma2

6. What is the formula for the perpendicular axis theorem?

Iz=Ix+Iy , where I’s are the moments of inertia in Z, X and Y axes respectively.

7. What is the formula for the parallel axis theorem?

I=ICOM+Mh2, h is the perpendicular distance between the axis passing through the COM and the axis about which we have to find the moment of inertia. Both of these axes of rotation must be parallel to each other.