Sequences and Series - Topics, Formula, Books, FAQs

Sequences and Series in Mathematics

Almost everything in nature, like the arrangement of flower petals, patterns in animal skins, branching in plants, snowflakes, honeycombs, and even human DNA fragments, follows a certain pattern. Going through this article, you will learn the sequence and series formula, important concepts from the sequence and series class 11, and how to apply them in various problems. This chapter plays an important role in solving problems related to progressions and is widely used in science, economics, and competitive exams.

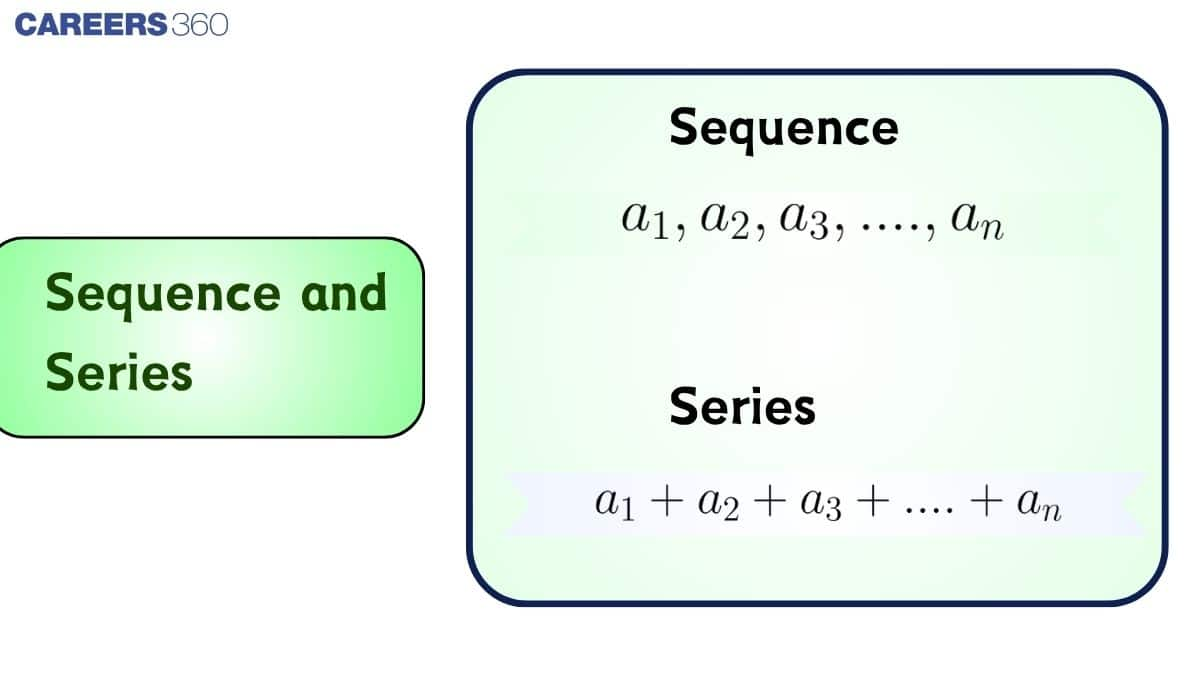
What is Sequence?

A sequence is a list of numbers written in a specific order where each term follows a definite pattern. The number of terms in a sequence can be either finite or infinite. The total number of terms is called the length of the sequence.

Examples of Sequence

• $1, 2, 3, 4, 5, \dots$

• $1, 4, 9, 16, \dots$

• $\frac{1}{3}, \frac{1}{4}, \frac{1}{5}, \frac{1}{6}, \dots$

These are examples covered under sequence and series class 11 where the focus is on identifying patterns and formulating the general term.

Types of Sequence – Sequence and Series

Sequences are an important part of mathematics and are widely used in various problems in the sequence and series class 11, sequence and series formulas, and class 11 maths sequence and series. Based on the number of terms and the nature of patterns, sequences are classified into several types.

Based on the Number of Terms

Finite Sequence

A sequence is called a finite sequence if it has a definite number of terms. The number of terms is called the length of the sequence.
Example:
2,4,6,82, 4, 6, 82,4,6,8
Here, the sequence has four terms, so it is a finite sequence.

Infinite Sequence

A sequence is called an infinite sequence if it continues indefinitely without ending. This is indicated by dots ($\dots$) at the end of the sequence.
Example:
2,4,6,8,10,…2, 4, 6, 8, 10, \dots2,4,6,8,10,…
Here, the sequence goes on forever, so it is an infinite sequence.

Based on the Characteristics of Terms

Arithmetic Progression (AP) – Sequence and Series

An arithmetic progression is a sequence in which the difference between any two consecutive terms is constant. This difference is known as the common difference and is denoted by $d$. The first term is denoted by $a$.

General form: $a, \; a+d, \; a+2d, \; a+3d, \dots$

First Term ($a$): The first term is the starting element of the sequence. It is denoted by $a$.

Example: In the sequence $5, \; 10, \; 15, \; 20, \dots$ the first term $a$ is $5$.

Common Difference ($d$): The common difference $d$ is the fixed value added to each term to obtain the next term.

Example: For the sequence $4, 10, 16, 22, \dots$ the common difference is $d = 10 - 4 = 6 = 16 - 10 = 6 = 22 - 16 = 6$

Examples of Arithmetic Progression:

Find the first term and common difference of the sequence: $2, 5, 8, 11, 14, 17, \dots$

The first term is $a = 2$ and the common difference is $d = 5 - 2 = 3$

Check if the sequence $4, 10, 16, 22, 28, \dots$ is an arithmetic progression.

The common difference is $d = 10 - 4 = 6 = 16 - 10 = 6 = 22 - 16 = 6 = 28 - 22 = 6$

Since the difference is constant, it is an AP.

Geometric Progression (GP) – Sequence and Series

A geometric progression is a sequence in which each term after the first is obtained by multiplying the previous term by a constant called the common ratio, denoted by $r$. The first term is denoted by $a$.

General form:
$a, ar, ar^2, ar^3, \dots$

Common Ratio ($r$):
The common ratio $r$ is the factor by which each term is multiplied to get the next term.
$r = \frac{a_2}{a_1} = \frac{a_3}{a_2} = \dots = \frac{a_n}{a_{n-1}}$

Examples of Geometric Progression:

1. $2, 6, 18, 54, \dots$ Here, $a = 2$ and $r = 3$.

2. $4, 2, 1, \frac{1}{2}, \frac{1}{4}, \dots$ Here, $a = 4$ and $r = \frac{1}{2}$.

3. $-5, 5, -5, 5, \dots$ Here, $a = -5$ and $r = -1$.

Harmonic Progression (HP) – Sequence and Series

A harmonic progression is formed by taking the reciprocals of an arithmetic progression. All terms must be non-zero, and the sequence is defined as the sequence of reciprocals of the corresponding AP.

Definition:
A sequence $a_1, a_2, a_3, \dots, a_n, \dots$ is a harmonic progression if
$\frac{1}{a_1}, \frac{1}{a_2}, \frac{1}{a_3}, \dots, \frac{1}{a_n}, \dots$ forms an arithmetic progression.

Example: $\frac{1}{2}, \frac{1}{5}, \frac{1}{8}, \frac{1}{11}, \dots$
The reciprocals form the arithmetic sequence $2, 5, 8, 11, \dots$, hence, this is an HP.

Important Notes:

• No term in an HP can be zero.

• The general form is: $\frac{1}{a}, \frac{1}{a+d}, \frac{1}{a+2d}, \dots$
  where $a$ is the first term and $d$ is the common difference of the corresponding AP.

What is a Series?

A series is the sum of all terms in a sequence. When we add or subtract the terms of a sequence, the result is called a series, and it is denoted by $S_n$.

Series Formula

If the sequence is $a_1, a_2, a_3, \dots, a_n$, then the series is:

$S_n = a_1 + a_2 + a_3 + \dots + a_n = \sum_{r=1}^{n} a_r$

This formula is a key part of the sequence and series formulas class 11, which helps in solving problems where the summation of terms is required.

Types of Series

Based on the number of terms, there are two types of series:

1. Finite series
2. Infinite Series

Finite Series

If the sequence has only a finite number of terms, then the series is called a finite sequence. e.g. $2+4+6+8$

Infinite Series

If a sequence has three dots at the end, then it indicates the list never ends. It has an infinite number of terms. Then, the series is said to be an infinite series.

$
\text { E.g., } 2+4+6+8+10+\ldots
$

Based on the characteristics of the terms, the types of series are,

1. Arithmetic Series
2. Geometric Series
3. Harmonic Series

Arithmetic Series

The sum of terms of an arithmetic sequence is called the arithmetic series. The sum of the first $n$ terms of an arithmetic series is $S_n=\frac{n}{2}(2 a+(n-1) \cdot d)$ where $a$ is the first term and $d$ is the common difference.

Geometric Series

The sum of terms of a geometric sequence is called the geometric series. The sum of the first $n$ terms of a geometric series is $S_n=a \cdot \frac{1-r^n}{1-r} \quad($ for $r \neq 1)$ where $a$ is the first term and $d$ is the common difference.

Harmonic Series

The sum of the terms in a harmonic sequence is called the harmonic series. The sum of the first $n$ terms of a harmonic series is $S_n=1+\frac{1}{2}+\frac{1}{3}+\cdots+\frac{1}{n}$.

Sequence and Series Formulae

Sequence and series class 11 formulas include the terms of the sequences and the sum of the first n terms of the series.

Sequence Formulae

Sequence formulas include formulas of arithmetic progression, geometric progression and harmonic progression.

Arithmetic Progression

In an Arithmetic Progression (AP), several terms and notations are commonly used to describe and calculate the sequence. These terms and notations are as follows:

The number of terms (n): As the name suggests, the number of terms of an AP is the total number of terms present in the progression or in the sequence. It is usually denoted as $n$.

For example, $1, 2, 3, 4, 5$ is in AP where the number of terms ($n$) is $5$.

The general term of an AP ($t_n$): The general term or the nth term of an arithmetic sequence can be expressed in two ways i.e. the $n$th term of an AP from the beginning and the nth term of an AP from the last. We will discuss these two ways below.

The nth term of an AP from the beginning: The general term of the nth term from the beginning of an AP where the first term is $a$, the common difference is $d$, and the number of terms is $n$ is given by the formula:

$t_n = a + (n - 1)d$.

The nth term of an AP from the last: The nth term of an AP from the last, where the last term is $l$, the common difference is $d$, and the number of terms is $n$, is given by the formula:

$t_n = l - (n - 1)d$.

The nth term of an AP if the mth term is given, but the first term is not given: The mth term of an AP is given.

So, $t_m=a+(m-1)d$, where $a$ is the first term and $d$ is the common difference.

Since the first term is not given, we need to find the first term in terms of the given mth term.

So, $a = t_m - (m-1)d$

Now, for the nth term,

$t_n = a+(n-1)d = t_m - (m-1)d + (n-1)d = t_m - md + d + nd - d = t_m + (n-m)d$

Therefore, $t_n = t_m + (n - m)d$ is the required nth term of an AP when the mth term is given, but the first term is not given.

Arithmetic Mean

The arithmetic mean of a set of $n$ numbers is calculated by summing all the numbers and then dividing by $n$. For a set of $n$ positive integers $a_1, a_2, a_3, a_4$, $\dots$ $a_n$.

Arithmetic mean $=\frac{a_1+a_2+a_3+a_4+\ldots \ldots .+a_n}{n}$

For example, AM of 2, 4, 6, 8, 10 is $\frac{2 + 4 + 6 + 8 + 10}{5} = 6$.

Geometric Progression

General Term of a GP: If ' $a$ ' is the first term and ' $r$ ' is the common ratio, then
$
\begin{aligned}
& a_1=a=a r^{1-1}\left(1^{\text {st }} \text { term }\right) \\
& a_2=a r=a r^{2-1}\left(2^{\text {nd }} \text { term }\right) \\
& a_3=a r^2=a r^{3-1}\left(3^{\text {rd }} \text { term }\right) \\
& \cdots \\
& \cdots \\
& a_n=a r^{n-1}\left(\mathrm{n}^{\text {th }} \text { term }\right)
\end{aligned}
$

So, the general term or $\mathrm{n}^{\text {th }}$ term of a geometric progression is $a_n=a r^{n-1}$

Geometric Mean

The geometric mean of $n$ numbers is the $n$th root of the product of the numbers.

For a set of $n$ positive integers $a_1, a_2, a_3, a_4$,$ $\dots\mathrm{a}_{\mathrm{n}}$

Geometric mean $=\sqrt[n]{a_1 \times a_2 \times a_3 \times a_4 \times \ldots \ldots . \times a_n}$

Example: Geometric mean of $2, 4, 8,$ and $16=$ $\sqrt[4]{2 \times 4 \times 8 \times 16}=\sqrt[4]{1024}=5.66$

Harmonic Progression

The general term of a Harmonic Progression: The nth term or general term of an H.P. is the reciprocal of the nth term of the corresponding A.P. Thus, if $a_1, a_2, a_3, \ldots \ldots, a_n$ is an H.P. and the common difference of corresponding A.P. is d, i.e. $d=\frac{1}{a_n}-\frac{1}{a_{n-1}}$, then the nth term of corresponding AP is $\frac{1}{a_1}+(n-1) d$, and hence, the
general term or nth term of an H.P. is given by

$
a_n=\frac{1}{\frac{1}{a_1}+(n-1) d}
$

Harmonic Mean

The Harmonic Mean is the reciprocal of the average of the reciprocals of a given set of numbers. For a set of $n$ positive integers $a_1, a_2, a_3, a_4$, $\dots$ .$a_n$

$
\text { Harmonic mean }=\frac{n}{\frac{1}{a_1}+\frac{1}{a_2}+\frac{1}{a_3}+\frac{1}{a_4}+\ldots \ldots . .+\frac{1}{a_n}}
$

Series formulas

Series formulas include the sum of the first n terms in the arithmetic series, geometric series and the harmonic series.

Sum of n terms in arithmetic series: The sum of the first $n$ terms of an arithmetic series is $S_n=\frac{n}{2}(2 a+(n-1) \cdot d)$ where $a$ is the first term and $d$ is the common difference.

Sum of n terms in geometric series: The sum of the first $n$ terms of a geometric series is $S_n=a \cdot \frac{1-r^n}{1-r} \quad($ for $r \neq 1)$ where $a$ is the first term and $d$ is the common difference.

Sum of n terms in harmonic series: There is no general formula for the sum of the first $n$ terms that are in H.P. The sum of the first $n$ terms of a harmonic series is $S_n=1+\frac{1}{2}+\frac{1}{3}+\cdots+\frac{1}{n}$.

Difference Between Sequence and Series

The difference between sequence and series is given below:

Sequences and Series in Mathematics: Solved Previous Year Questions

Question 1:

If $\mathbf{a}_1, \mathbf{a}_2, \mathbf{a}_3 \ldots$. is an A.P. and $S_r=a_r+a_{r+1}+\ldots+a_{r+n-1}$, then the value of $\sum_{r=1}^n S_r$ is:

Solution:

$\begin{aligned} & \mathrm{S}_{\mathrm{r}}=\frac{\mathrm{n}}{2}\left(\mathrm{a}_{\mathrm{r}}+\mathrm{a}_{\mathrm{r}+\mathrm{n}-1}\right) \\ = & \frac{\mathrm{n}}{2}\left[\mathrm{a}_1+(\mathrm{r}-1) \mathrm{d}+\mathrm{a}_1+(\mathrm{r}+\mathrm{n}-2) \mathrm{d}\right] \\ = & \frac{\mathrm{n}}{2}\left[\mathrm{a}_1+\mathrm{a}_{\mathrm{n}}+2(\mathrm{r}-1) \mathrm{d}\right] \\ & \Sigma \mathrm{S}_{\mathrm{r}}=\frac{\mathrm{n}}{2}\left[n \mathrm{na}_1+\mathrm{na} \mathrm{a}_{\mathrm{n}}+2 \mathrm{~d} \sum_{\mathrm{r}=1}^{\mathrm{n}}(\mathrm{r}-1)\right] \\ = & \frac{\mathrm{n}}{2}\left[n a_1+\mathrm{na} \mathrm{n}_{\mathrm{n}}+2 \mathrm{~d} \frac{(\mathrm{n}-1) \mathrm{n}}{2}\right] \\ = & \frac{\mathrm{n}^2}{2}\left[\mathrm{a}_1+\mathrm{a}_{\mathrm{n}}+(\mathrm{n}-1) \mathrm{d}\right] \\ = & \frac{\mathrm{n}^2}{2}\left[2 \mathrm{a}_{\mathrm{n}}\right]=\mathrm{n}^2 \mathrm{a}_{\mathrm{n}}\end{aligned}$

Hence, the answer is $\mathrm{n}^2 \mathrm{a}_n$.

Question 2:

If $\sum_{r=0}^{n+4} a_r x^r=(x+1)^n\left(1+x^2\right)^2$ and $a_1, a_2, a_3$ are in AP, then n is

Solution:

$\begin{aligned} & \quad \sum_{r=0}^{n+4} a_r x^r=(x+1)^n\left(x^4+2 x^2+1\right) \\ & \mathrm{a}_1={ }^{\mathrm{n}} \mathrm{C}_1, \mathrm{a}_2={ }^{\mathrm{n}} \mathrm{C}_2+2^{\mathrm{n}} \mathrm{C}_0, \mathrm{a}_3={ }^{\mathrm{n}} \mathrm{C}_3+2^{\mathrm{n}} \mathrm{C}_1 \\ & \Rightarrow \quad \mathbf{a}_1+\mathbf{a}_3=2 \mathbf{a}_2 \\ & \Rightarrow \quad n+\frac{n(n-1)(n-2)}{6}+2 n=n(n+1)+4 \\ & \quad \Rightarrow \quad n^3-3 n^2+2 n+18 n=6 n^2-6 n+24 \\ & \Rightarrow \quad n^3-9 n^2+26 n-24=0 \\ & \Rightarrow \quad(n-2)(n-3)(n-4)=0 \\ & \mathrm{n}=2,3,4\end{aligned}$

Hence, the answer is 2, 3, and 4.

Question 3:

If $t_n$ denotes the $n^{\text {th }}$ term of the series $2+3+6+11+18+\ldots$ then $t_{50}$ is:

Solution:

$
\begin{aligned}
& S_n=2+3+6+11+18+\ldots+t_{50}(\text { Using method of difference) } \\
& S_n=0+2+3+6+11+18+\ldots+t_{50} \\
& S_n-S_n=(2-0)+(3-2)+(6-3)+(11-6)+(18-11)+\ldots-t_{50} \\
& t_{50}=2+[1+3+5+7+\ldots \text { upto } 49 \text { terms }] \\
& t_{50}=2+\frac{49}{2}[2+(49-1) 2] \\
& t_{50}=2+49^2
\end{aligned}
$
Hence, the answer is $49^2+2$.

Question 4:

Let $S_n$ denote the sum of the first n terms of an A.P. If then $S_{3 \mathrm{n}}: S_{\mathrm{n}}$ is equal to:

Solution:

Given $S_{2 n}=3 S_n$

$
\begin{aligned}
& S_n=\frac{n}{2}[2 a+(n-1) d] \\
& S_{2 n}=\frac{2 n}{2}[2 a+(2 n-1) d] \\
& S_{2 n}=3 S_n \\
& n[2 a+(2 n-1) d]=\frac{3 n}{2}[2 a+(n-1) d] \\
& 4 a+4 n d-2 d=6 a+3 n d-3 d \\
& 2 a=n d+d \\
& S_{3 n}=\frac{3 n}{2}[2 a+(3 n-1) d]=\frac{3 n}{2}[(n+1) d+(3 n-1) d]=6 n^2 d \\
& S_n=\frac{n}{2}[2 a+(n-1) d]=\frac{n}{2}[(n+1) d+(n-1) d]=n^2 d \\
& \frac{S_{3 n}}{S_n}=\frac{6 n^2 d}{n^2 d}=6
\end{aligned}
$

Hence, the correct answer is 6.

Question 5:

The third term of G.P. is 4. The product of its first 5 terms is:

Solution:

The third term of G.P is given $T_3=4$

$
\begin{aligned}
& T_n=a r^{n-1} \\
& a r^2=4
\end{aligned}
$
Product of first 5 terms

$
\begin{aligned}
& =(a)(a r)\left(a r^2\right)\left(a r^3\right)\left(a r^4\right) \\
& =\left(a^5 r^{10}\right)=\left(a r^2\right)^5 \\
& =4^5=1024
\end{aligned}
$

Hence, the correct answer is $4^5$.

List of topics related to sequence and series according to NCERT/JEE Mains

This section covers all important sequences and series topics as per the NCERT and JEE MAIN syllabus. You’ll find a complete list to help you focus on the most relevant concepts for class 11 sequence and series questions and formulas.

Sequences and Series in Different Exams

The chapter on Sequences and Series is an important part of Class 11 Mathematics and is frequently tested in school exams as well as competitive examinations. It focuses on identifying patterns and finding sums, which are essential skills in higher mathematics. The table below highlights the exam-wise focus areas, commonly asked topics, and preparation strategies to help students prepare effectively.

Important Books for Sequences and Series

Find the best books recommended for mastering sequences and series for class 11 and JEE MAIN preparation. These books cover sequence and series formulas, examples, and solved problems to strengthen your understanding.

NCERT Resources for Sequences and Series

Explore NCERT sequence and series class 11 notes, solutions, and formulas. These resources are essential for building a strong foundation in sequence and series concepts and solving related problems.

• NCERT Maths Notes for Class 11th Chapter 9 Sequence and Series
• NCERT Maths Solutions for Class 11th Chapter 9 Sequence and Series
• NCERT Maths Exemplar Solutions for Class 11th Chapter 9 Sequence and Series

NCERT Subjectwise Resources

Explore NCERT class 11 notes and solutions for different subjects. These resources are essential for building a strong foundation in various concepts and solving related problems.

Practice Questions based on Sequence and Series

Practice questions based on Sequence and Series help students strengthen their understanding of patterns in numbers and improve problem-solving skills. Regular practice enhances speed and accuracy, which is essential for scoring well in exams.

Conclusion

Sequences and Series help students understand patterns, progressions, and numerical relationships in mathematics. A strong command of formulas for AP, GP, and special series makes problem-solving easier and faster. With regular practice and clear conceptual understanding, students can confidently handle questions in board exams and competitive examinations.