Derivation of Centripetal Acceleration - Detailed Guide
Imagine driving around a curve and feeling a pull outward—that's centripetal acceleration in action, a key concept in circular motion. It helps explain how Forces work on a vehicle during turns. Understanding this can make driving safer, especially when navigating curves at higher speeds. In this report, we explore the role of centripetal acceleration, factors that affect it like speed and friction, and practical tips for handling turns. In this article we will be studying centripetal acceleration is, centripetal acceleration formula, centripetal acceleration derivation, centripetal force, define centripetal acceleration, direction of centripetal acceleration and centrifugal acceleration formula. Define Centripetal Acceleration.
This Story also Contains
- Define Centripetal Acceleration and Derive an Expression
- Centripetal Acceleration Formula:
- Centripetal Acceleration Derivation
- Centripetal Force
- Centripetal Force Derivation
- Centrifugal Force Formula Derivation
- Centripetal Force vs centrifugal Force
- Problem-Solving Strategy
- Weitage of Centripetal Acceleraion in Diffrent Exam
This concept, covered in Class 11 Physics under circular motion, derivation of centripetal acceleration is essential for board exams and competitive exams like JEE Main, NEET, BITSAT, and others. Between 2013 and 2023, five questions on this topic have appeared in JEE Main.
Define Centripetal Acceleration and Derive an Expression
Centripetal acceleration is the acceleration of a body that is travelling across a circular path. When a body undergoes a circular motion, its direction constantly changes and thus its velocity changes (velocity is a vector quantity) which produces an acceleration. The centripetal acceleration ac is given by the square of speed v divided by the distance "r".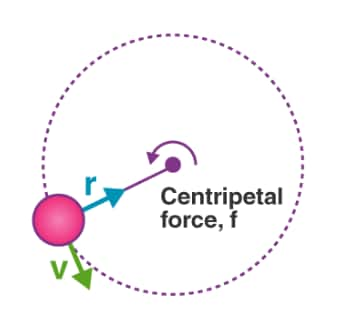

Here, a question arises that why is it called centripetal acceleration while the speed of rotation remains constant?
The question is valid. Now we’re providing you with the answer to the query, and it is because speed is a scalar quantity. At the same time, in rotational motion, there is no actual speed of rotation because it includes direction as well; instead, there is rotational velocity(vector quantity). Hence, velocity variation leads to the term named centripetal acceleration.
Centripetal Acceleration Formula:
$$
a_c=\frac{v^2}{r}
$$
where:
-.$a_c$ is the centripetal acceleration,
- $v$ is the velocity of the object,
- $r$ is the radius of the circular path.
This is the required Centripetal acceleration Formula.
- Centripetal acceleration unit: metre per second squared (m/s2).
- Centripetal acceleration Dimensional formula:
The dimensional formula of centripetal acceleration is given as:
Dimensional formula of radius = M0L1T0
Dimensional formula of Velocity = M0L1T-1
Substituting the above in the equation of the centripetal formula a= v2/r, we get:
Dimensional formula of Centripetal acceleration = M0L1T-2
Note: The force causing this acceleration is also directed towards the centre of the circle and is named centripetal force.
Also read -
Centripetal Acceleration Derivation
Consider a body of mass ‘m’ moving on the circumference of a circle of radius ‘r’ with a velocity ‘v’. A force F is then applied to the body. And this force is given by
F = ma
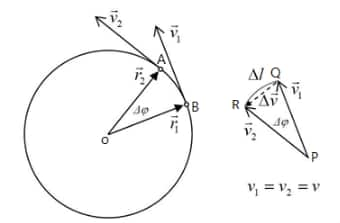

Where, a= acceleration which is given by the rate of change of velocity with respect to time.
Consider the triangles $\triangle O A B$ and $\triangle P Q R$, then:
$$
\Delta v_{A B}=\frac{v}{r}
$$
Clearly, from the diagram (not shown here), the arc length $A B=v \Delta t$.
Now, we have:
$$
\frac{\Delta v}{v \Delta t}=\frac{v}{r}
$$
Simplifying this:
$$
\frac{\Delta v}{\Delta t}=\frac{v^2}{r}
$$
Thus, the centripetal acceleration equation is:
$$
a=\frac{v^2}{r}
$$
Note: The direction of centripetal acceleration(& force) is towards the centre of the circle.
Now, let's move to another part of this article which is Centripetal Force
What is the direction of centripetal acceleration?
The direction of centripetal acceleration and also force is towards the centre of the circle.
Centripetal Force
It is the force that acts on a body undergoing circular motion and is directed towards the centre of the rotation(or the circle).
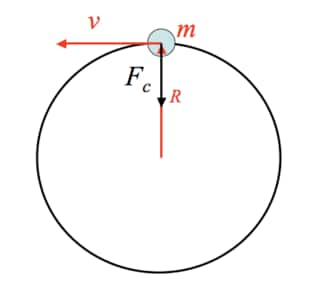

Centripetal Force Derivation
Centripetal force is the net force causing uniform circular motion.
According to Newton’s laws of motion,
The force is given by $F=m a$, where:
- $m$ is the mass of the body,
- $a$ is the acceleration of the body.
In the case of uniform circular motion, the acceleration is the centripetal acceleration, given by:
$$
a_c=\frac{v^2}{r}
$$
where:
- $v$ is the linear velocity of the object,
- $r$ is the radius of the circular path.
Now, using angular velocity $(\omega)$, centripetal acceleration can also be expressed as:
$$
a_c=r \omega^2
$$
where:
- $\omega$ is the angular velocity of the object,
- $r$ is the radius of the circular path.
Thus, the centripetal force formula using linear velocity is:
$$
F_c=\frac{m v^2}{r}
$$
Here:
- $F_c$ is the centripetal force,
- $m$ is the mass of the object,
- $v$ is the linear velocity,
- $r$ is the radius of the circular path.
The centripetal force formula in terms of angular velocity is given by:
$$
F_c=m r \omega^2
$$
where:
- $F_c$ is the centripetal force,
- $m$ is the mass of the object,
- $r$ is the radius of the circular path,
- $\omega$ is the angular velocity.
Thus, the expression for centripetal force in terms of angular velocity is:
$$
F_c=m r \omega^2
$$
Or,
We can say that $\frac{m v^2}{r}$is the formula of centripetal acceleration.
It is also known as angular centripetal force/derivation of centrifugal force class 11
Centrifugal Force Formula Derivation
After centripetal force derivation, now in this section, we are going to study centrifugal force derivation and its formula which is one of the important concepts for board exams.
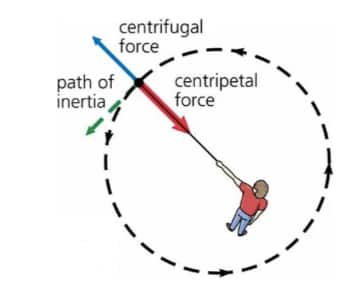

Centrifugal Force
$$
F=m \times \frac{v^2}{r}
$$
2. In terms of angular velocity $\omega$ :
$$
F=m \times \frac{(r \omega)^2}{r}=m \times r \omega^2
$$
Thus, the formula for centripetal force can be expressed as either:
$$
F=m \times \frac{v^2}{r}
$$
or
$$
F=m \times r \omega^2
$$
where $m$ is the mass, $v$ is the linear velocity, $r$ is the radius of the circular path, and $\omega$ is the angular velocity.
NOTE:
- For a particle in circular motion, the centripetal acceleration is:
$$
a_c=\frac{v^2}{r} \quad \text { or } \quad a_c=r \omega^2
$$
- The expression $m \omega^2 r$ represents the centripetal force.
- The formula for centrifugal force (which is equal in magnitude but opposite in direction to centripetal force) is:
$$
F=m \omega^2 r=\frac{m v^2}{r}
$$
Derive v= r ω
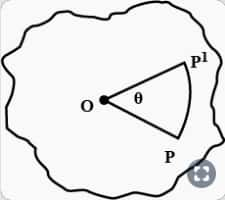

Let us consider a body rotating about an axis that is passing through O point and also perpendicular to the plane.
Let us suppose, P be the position of a particle inside the body. If the body rotates an angle 0 in a time ‘t’, the particle at which is at P reaches P′
The displacement along the circular path is:
$$
P P^{\prime}=r \theta
$$
The velocity is:
$$
v=\frac{P P^{\prime}}{t}=\frac{r \theta}{t}
$$
Since $\frac{\theta}{t}=\omega$ (angular velocity), we have:
$$
v=r \omega
$$
Using the relationship $v=r \omega$, we can write:
$$
v^2=(r \omega)^2=r^2 \omega^2
$$
Substitute $v^2$ into the acceleration formula:
$$
a=\frac{r^2 \omega^2}{r}
$$
Simplifying:
$$
a=r \omega^2
$$
\begin{aligned}
&\text { Thus, the centripetal acceleration can be expressed as: }\\
&a=\frac{v^2}{r}=r \omega^2
\end{aligned}
Also read
Centripetal Force vs centrifugal Force
In the previous section, we derive an expression for centripetal acceleration. A key concept that often comes to mind is the difference between centripetal and centrifugal forces. Below, we outline some of the fundamental differences between these two forces. Please review them carefully.
Centripetal Force:
Definition: Centripetal force is the force that acts on an object moving in a circular path, directed towards the centre of the circle. It keeps the object moving in a curved trajectory rather than in a straight line.
Nature: A real force that acts on the object.
Direction: Always directed towards the centre of the circular path.
Formulae: $F_c=\frac{m v^2}{r}$
Examples:
1. The tension in a string when swinging a ball in a circular motion.
2. The gravitational force acting on planets keeps them in orbit.
Centrifugal Force:
Definition: Centrifugal force is a pseudo or fictitious force that appears to act on an object when it is observed from a rotating reference frame. It seems to push the object away from the centre of rotation.
Nature: Not a real force; it is an apparent force observed in a non-inertial (rotating) frame of reference.
Direction: Directed away from the centre of the circular path, opposite to the direction of centripetal force.
Formula: Fcentrifugal =$-\frac{m v^2}{r}=-m r \omega^2$
Examples:
1. The sensation of being "thrown" outward when a car makes a sharp turn.
2. The apparent force felt by passengers in a spinning amusement park ride.
Recommended Topic Video
Problem-Solving Strategy
1. Identify the plane of circular motion.
2. Locate the centre of rotation and calculate the radius.
3. Make F.B.D.
4. Resolve force along the radial direction and along the direction perpendicular to it.
5. The net force along the radial direction is mass times the radial acceleration is no separate force like Tension, Weight, Spring force, Normal reaction, Friction etc. In fact any of these or their combination may play a role of centripetal force.
After going through the derivation of centripetal acceleration now you will able to solve questions based on this concept and this derivation is also very important for board exams.
Weitage of Centripetal Acceleraion in Diffrent Exam
| Exam | Weight of Centripetal Acceleration | Remarks |
| JEE Main and JEE Advanced | Moderate to High | Often tested in mechanics, rotational dynamics, and central forces problems. |
| NEET (Medical Entrance Exam) | Moderate | Related to biomechanics and motion in circular paths, but less focus compared to engineering exams. |
| CUET (Common University Entrance Test) | Low to Moderate | Tested in science-oriented courses; typically involves straightforward, application-based questions. |
| GATE (Graduate Aptitude Test in Engineering) | High | Critical in dynamics and machine theory, particularly in mechanical and civil engineering sections. |
| Class 12 Board Exams (CBSE/State Boards) | Moderate | Part of the physics syllabus in mechanics and circular motion chapters; direct formula-based questions. |
| Olympiads (National and International) | High | Deeply explored in challenging mechanics problems requiring advanced understanding and applications. |
Also, check-
Frequently Asked Questions (FAQs)
We start with the formula for centripetal force:
$$
F=\frac{m v^2}{r}
$$
Also, from Newton's 2nd law:
$$
F=m a
$$
We can express acceleration as:
$$
a=\frac{F}{m}
$$
Substituting the value of $F$ from the centripetal force equation:
$$
a=\frac{\frac{m v^2}{r}}{m}
$$
Simplifying it:
$$
a=\frac{v^2}{r}
$$
Thus, the centripetal acceleration is:
$$
a=\frac{v^2}{r}
$$
Proof:
1. $F=\frac{m v^2}{r}$ (using centripetal force)
2. $F=m a$ (using Newton's 2nd law)
We can rewrite this as:
3. $a=\frac{F}{m}$
Substituting the value of $F$ from equation (1) into equation (3):
4. $a=\frac{m v^2}{r} \div m$
Simplifying:
5. $a=\frac{v^2}{r}$
This is the required expression for acceleration in circular motion.
Centripetal acceleration is the acceleration of a body that is travelling across a circular path. When a body undergoes a circular motion, its direction constantly changes and thus its velocity changes (velocity is a vector quantity) which produces an acceleration.Derivation:
$F=\frac{m v^2}{r}$ (using centripetal force)
$F=m a$ (using Newton's second law)
We can rewrite this as:
$a=\frac{F}{m}$
Substituting the value of $F$
\$a=\frac{m v^2}{r} \div m$
$a=\frac{v^2}{r}$
Thus, the centripetal acceleration is given by $a=\frac{v^2}{r}$.
Derivation for centripetal force is given in this article.