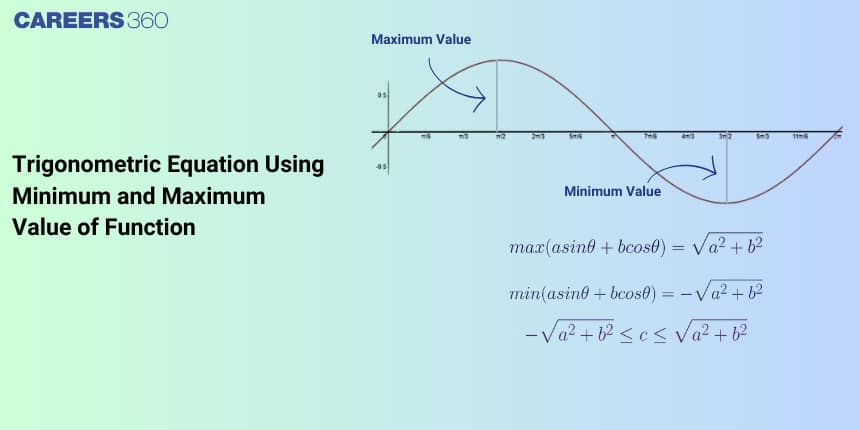
Trigonometric Equation using Minimum and Maximum value of Function

A trigonometric equation is any equation that contains trigonometric functions. Trigonometric equations are satisfied only for some values (finite or infinite in number) of the angles. The maximum and minimum value of the trignometric function gives us the range on which the value of the trignometric function lies.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. What are Trigonometric Equations?
2. What are the Minimum and Minimum Values of Trignometric Equations?
3. Maximum and Minimum Value of Trignometric Functions
4. Trigonometric Equation using Minimum and Maximum values of Trigonometric Functions
5. Steps to Solve Trigonometric Equation Using Minimum and Maximum Values of Trigonometric Functions

In this article, we will cover the concept of Solution of Trigonometric Equations. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), 12 questions have been asked on this topic, including one from 2021 and one from 2022.

What are Trigonometric Equations?

Trigonometric equations are, as the name implies, equations that involve trigonometric functions. Trigonometric equations are satisfied only for some values (finite or infinite in number) of the angles. A value of the unknown angle that satisfies the given trigonometric equation is called a solution or a root of the equation. For example, equation $2\sin x=1$ is satisfied by $x=\pi /6$ is the solution of the equation between $\circ$ and $\pi$. The solutions of a trigonometric equation lying in the interval $[0,\pi$ ) are called principal solutions.

What is the Solution of Trigonometric Equation?
The value of an unknown angle that satisfies the given trigonometric equation is called a solution or root of the equation.

For example, $2\sin \theta =1$, clearly $\theta ={30}^{\circ }$ satisfies the equation; therefore, ${30}^{\circ }$ is a solution of the equation. Now trigonometric equation usually has infinite solutions due to the periodic nature of trigonometric functions. So this equation also has $(360+30{)}^0,(720+30{)}^0,(-360+30{)}^0$, and so on, as its solutions.

Principal Solution

The solutions of a trigonometric equation that lie in the interval $[0,2\pi )$. For example, if $2\sin \theta =1$, then the two values of $\sin \theta$ between 0 and $2\pi$ are $\pi /6$ and $5\pi /6$.

Thus, $\pi /6$ and $5\pi /6$ are the principal solutions of equation $2\sin \theta =1$.

General Solution

As trigonometric functions are periodic, solutions are repeated within each period, so, trigonometric equations may have an infinite number of solutions. The solution consisting of all possible solutions of a trigonometric equation is called its general solution.

What are the Minimum and Minimum Values of Trignometric Equations?

The maximum and minimum values of trigonometric functions depend upon the range of the trigonometric functions. The minimum value of the trignometric function is the lowest value of the range and the maximum value is the highest value of the range.

Maximum and Minimum Value of Trignometric Functions

According to the domain and range, the maximum and minimum values of the trigonometric functions can be determined. The maximum and minimum values of trignometric functions are given below:

Trigonometric Equation using Minimum and Maximum values of Trigonometric Functions

Sometimes, we use the maximum and minimum values of trigonometric functions to solve trigonometric equations.

While solving the equation of the type $f(x)=g(x)$, and $x\in A$, we may come across a situation like

If $x\in A,f(x)(LHS)\le$ a and $g(x)(RHS)\ge a$, then the only condition under when LHS equals RHS: $f(x)=g(x)$ is when both equal $a$.

Steps to Solve Trigonometric Equation Using Minimum and Maximum Values of Trigonometric Functions

Step 1: Find the value of the trignometric equation.

Step 2: Corresponding to that value find the trignometric function value.

Let us go through some illustrations to understand this concept.

Illustrations 1

If $3\sin ax+4\cos x=7$, then the possible values of ' $a$ ' are
Here, we have given $3\sin ax+4\cos x=7$,
Now maximum value of LHS is $7(3+4=7)$, which occurs when $\sin \mathrm{ax}=1$ and $\cos x=1$

So LHS will equal RHS only when LHS is at its maximum value of 7 , which is possible only when $\sin \mathrm{ax}=1$ and $\cos x=1$

So,

$\begin{array}{rl}& \mathrm{ax}=(4\mathrm{n}+1)\frac{\pi }{2}\text{ and }\mathrm{x}=2\mathrm{m}\pi ,\mathrm{n},\mathrm{m}\in \mathbb{I}\\ & \frac{(4\mathrm{n}+1)\pi }{2\mathrm{a}}=2\mathrm{m}\pi \\ & \mathrm{a}=\frac{(4\mathrm{n}+1)}{4\mathrm{m}},\mathrm{n},\mathrm{m}\in \mathbb{I}\end{array}$

Illustrations 2

$\sin (x)+\cos (x)=2$
Now the maximum value of LHS is 2 , which occurs when $\sin (x)=1$ and $\cos (x)=1$

So now we have to solve a system of simultaneous equations: $\sin (x)=1$ and $\cos (x)=1$

But we know that when $\sin (x)=1$, then $\cos (x)$ can take only one of the following values

$\sqrt{1-{\sin }^{2}x}\text{ or }-\sqrt{1-{\sin }^{2}x}\text{, i.e. }0\text{ only }$
So no value of $x$ exists which satisfies both the equations $\sin (x)=1$ and $\cos (x)=1$ simultaneously

So the given equation has no solution

Recommended Video Based on Trigonometric Equations Using Minimum and Maximum Values of Function:

Solved Examples Based on Trigonometric Equations using Minimum and Maximum values of Function

If ${\cos }^2{\theta }_1+{\cos }^2{\theta }_2+{\cos }^2{\theta }_3=0$, then which of the following is the possible value of $\sin {\theta }_1$ $+\sin {\theta }_2+\sin {\theta }_3$
1) $3$
2) $-3$
3) $-1$
4) All of above

Solution

$\begin{array}{rl}& {\cos }^2{\theta }_1+{\cos }^2{\theta }_2+{\cos }^2{\theta }_3=0\\ & {\theta }_1={\theta }_2={\theta }_3=\pm \frac{\pi }{2}\end{array}$

so possible values of $\sin {\theta }_1+\sin {\theta }_2+\sin {\theta }_3$ are $\{-3,3,1,-1\}$
Hence, the answer is the option 4.

Example 2: The number of solutions of ${\sin }^{7}x+{\cos }^{7}x=1,\mathrm{x}\in [0,4\pi ]$ is equal to
[JEE MAINS 2021]
Solution
We know that

$\begin{array}{rl}& {\sin }^{7}x⩽{\sin }^{2}x⩽1\text{ and }{\cos }^{7}x⩽{\cos }^{2}x⩽1\\ & \therefore {\sin }^{7}x+{\cos }^{7}x⩽{\sin }^{2}x+{\cos }^{2}x\\ & \Rightarrow {\sin }^{7}x+{\cos }^{7}x⩽1\end{array}$

Equality is possible only when,

$\begin{array}{rl}& {\sin }^{7}x={\sin }^{2}x\text{ and }{\cos }^{7}x={\cos }^{2}x\\ & \Rightarrow \sin x=0,1\text{ and }\cos x=0,1\\ & \Rightarrow (\sin x=0\text{ and }\cos x=1)\text{ or }(\cos x=0,\sin x=1)\\ & \Rightarrow x=0,2\pi ,4\pi ,x=\frac{\pi }{2},\frac{5\pi }{2}\end{array}$

So, there are 5 solutions.

Hence, the answer is 5.

Example 3: How many roots of equation $x\cos x=1$ are possible?
Solution: Trigonometric Equation using Minimum and Maximum value of Function

Sometimes, we use the maximum and minimum values of trigonometric functions to solve trigonometric equations.

While solving equations of the type $f(x)=g(x)$, and $x\in A$, we may come across the situation like

If $x\in A,f(x)\le$ a and $g(x)\ge a$, then $f(x)=g(x)=a$.

$\begin{array}{c}x\cos x=1\\ \cos x=\frac{1}{x}=y\\ \text{ as }x\to \mathrm{\infty },y\to 0^{+}\\ \text{as }x\to -\mathrm{\infty },y\to 0^{-}\end{array}$

The graph is given by

By graph, we can say that it has infinitely many solutions

Hence the answer is infinite.

Example 4: Find the minimum value of $16{\sec }^2\mathrm{\Theta }+9{\csc }^2\mathrm{\Theta }+2$

Solution: Minimum value of

$a{\sec }^2\mathrm{\Theta }+b{\csc }^2\mathrm{\Theta }=(\sqrt{a}+\sqrt{b}{)}^2$

Minimum value of

$16{\sec }^2\mathrm{\Theta }+9{\csc }^2\mathrm{\Theta }=(\sqrt{16}+\sqrt{9}{)}^2=49$

Now, the minimum value of $16{\sec }^2\mathrm{\Theta }+9{\csc }^2\mathrm{\Theta }+2$

$=49+2=51$

Hence, the answer is 51.

Example 5: Find the maximum value of $8^{\sin \mathrm{\Theta }}\times {16}^{\cos \mathrm{\Theta }}$.
Solution

$\begin{array}{rl}& 8^{\sin \mathrm{\Theta }}\times {16}^{\cos \mathrm{\Theta }}=2^{3\sin \mathrm{\Theta }}\times 2^{4\cos \mathrm{\Theta }}\\ & =2^{3\sin \mathrm{\Theta }+4\cos \mathrm{\Theta }}\end{array}$

Maximum value of $a\sin \mathrm{\Theta }+b\cos \mathrm{\Theta }=\sqrt{a^2+b^2}$
Maximum value of $3\sin \mathrm{\Theta }+4\cos \mathrm{\Theta }=\sqrt{3^2+4^2}$

$\begin{array}{rl}& =\sqrt{9+16}\\ & =5\end{array}$

maximum value of $8^{\sin \mathrm{\Theta }}\times {16}^{\cos \mathrm{\Theta }}$

$\begin{array}{rl}& =2^5\\ & =32\end{array}$

Hence, the answer is $32.$